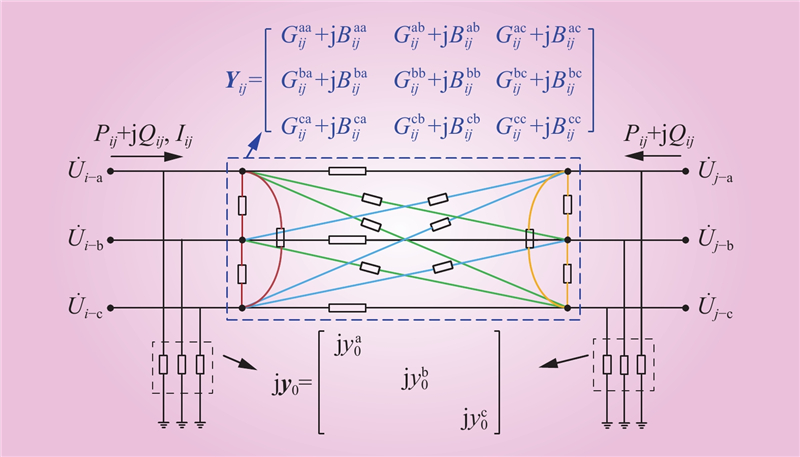
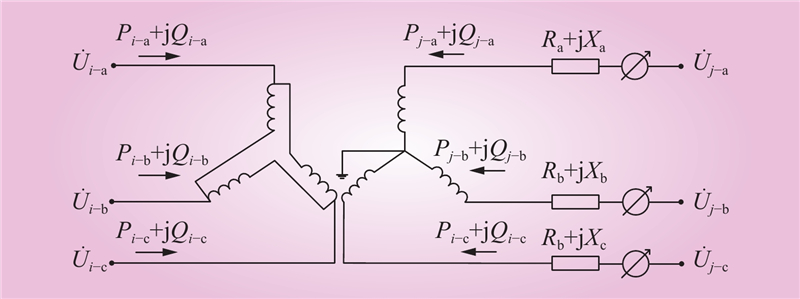
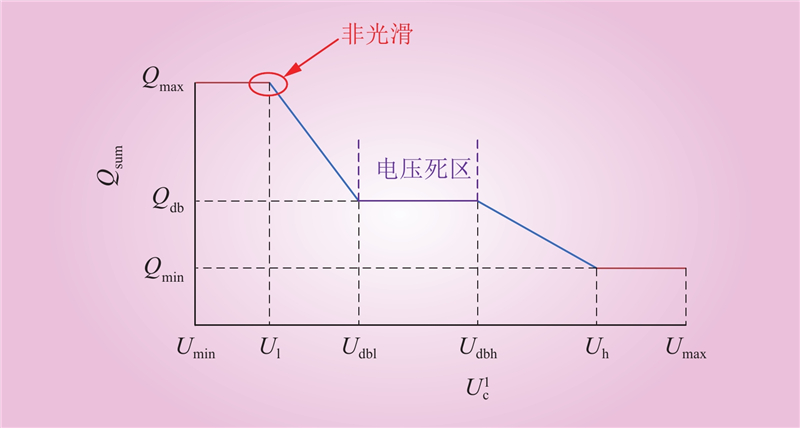
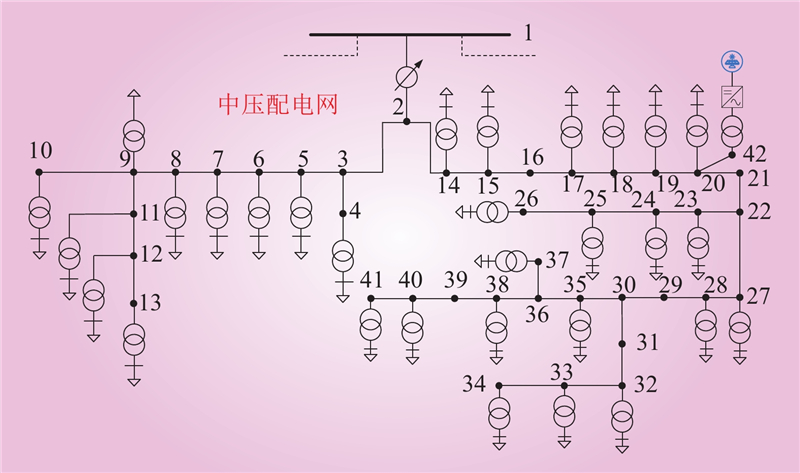
| 1 |
江木, 孙云超, 刘巨. 10 kV配电网理论线损计算方法及应用对比[J]. 供用电, 2019, 36 (11): 78- 83.
|
|
JIANG Mu, SUN Yunchao, LIU Ju. Theoretical line loss calculation method and application comparison for 10 kV distribution network[J]. Distribution & Utilization, 2019, 36 (11): 78- 83.
|
| 2 |
阮琪, 杨超, 王涵锋. 不同密度采集数据的配电网线损连续计算方法[J]. 电工材料, 2023, (6): 90- 94.
|
|
RUAN Qi, YANG Chao, WANG Hanfeng. Line loss calculation method for distribution network considering collected data of different densities[J]. Electrical Engineering Materials, 2023, (6): 90- 94.
|
| 3 |
丁心海, 罗毅芳, 刘巍, 等. 改进配电网线损计算方法的几点建议[J]. 电力系统自动化, 2001, 25 (13): 57- 60.
DOI
|
|
DING Xinhai, LUO Yifang, LIU Wei, et al. Proposalson improving the current methods for calculating line lossesof distribution network[J]. Automation of Electric Power Systems, 2001, 25 (13): 57- 60.
DOI
|
| 4 |
宋智强, 黄耀辉, 赵化时, 等. 含多类型直流的交直流混联电网潮流计算方法适用性分析[J]. 电力自动化设备, 2023.
|
|
SONG Zhiqiang, HUANG Yaohui, ZHAO Huashi, et al. Applicability analysis of classical power flow calculation methods for AC/DC hybrid power systems with multi-type DC links[J]. Electric Power Automation Equipment, 2023.
|
| 5 |
王磊, 张建宾, 余昆, 等. 基于典型负荷曲线的配电网线损计算方法研究[J]. 智慧电力, 2020, 48 (3): 124- 130.
DOI
|
|
WANG Lei, ZHANG Jianbin, YU Kun, et al. Calculation method of distribution line loss based on typical load curve[J]. Smart Power, 2020, 48 (3): 124- 130.
DOI
|
| 6 |
马喜平, 贾嵘, 梁琛, 等. 高比例新能源接入下电力系统降损研究综述[J]. 电网技术, 2022, 46 (11): 4305- 4315.
|
|
MA Xiping, JIA Rong, LIANG Chen, et al. Review of researches on loss reduction in context of high penetration of renewable power generation[J]. Power System Technology, 2022, 46 (11): 4305- 4315.
|
| 7 |
王成山, 李鹏. 分布式发电、微网与智能配电网的发展与挑战[J]. 电力系统自动化, 2010, 34 (2): 10- 14, 23.
|
|
WANG Chengshan, LI Peng. Development and challenges of distributed generation, the micro-grid and smart distribution system[J]. Automation of Electric Power Systems, 2010, 34 (2): 10- 14, 23.
|
| 8 |
田阳, 李志兵, 田宇, 等. 高比例新能源接入下快速真空断路器容性开合能力提升研究[J]. 电网技术, 2024, 48 (6): 2670- 2679.
|
|
TIAN Yang, LI Zhibing, TIAN Yu, et al. Research on the improvement of capacitive opening and closing ability of fast circuit breaker under high proportion of new energy access[J]. Power System Technology, 2024, 48 (6): 2670- 2679.
|
| 9 |
章博, 刘晟源, 林振智, 等. 高比例新能源下考虑需求侧响应和智能软开关的配电网重构[J]. 电力系统自动化, 2021, 45 (8): 86- 94.
|
|
ZHANG Bo, LIU Shengyuan, LIN Zhenzhi, et al. Distribution network reconfiguration with high penetration of renewable energy considering demand response and soft open point[J]. Automation of Electric Power Systems, 2021, 45 (8): 86- 94.
|
| 10 |
康重庆, 杜尔顺, 郭鸿业, 等. 新型电力系统的六要素分析[J]. 电网技术, 2023, 47 (5): 1741- 1750.
|
|
KANG Chongqing, DU Ershun, GUO Hongye, et al. Primary exploration of six essential factors in new power system[J]. Power System Technology, 2023, 47 (5): 1741- 1750.
|
| 11 |
KIM J, SEOK J K, MULJADI E, et al. Adaptive Q–V scheme for the voltage control of a DFIG-based wind power plant[J]. IEEE Transactions on Power Electronics, 2016, 31 (5): 3586- 3599.
DOI
|
| 12 |
巨云涛, 王健凯, 陈希, 等. 计及详细源荷模型的三相独立微电网连续潮流计算方法[J]. 电网技术, 2022, 46 (2): 718- 727.
|
|
JU Yuntao, WANG Jiankai, CHEN Xi, et al. Continuous power flow calculation method for three-phase isolated microgrid considering detailed source and load models[J]. Power System Technology, 2022, 46 (2): 718- 727.
|
| 13 |
肖峻, 周玉鹏, 鲍震宇. 配电系统优化问题建模中的潮流模型选择方法[J]. 电力系统自动化, 2023, 47 (15): 170- 178.
DOI
|
|
XIAO Jun, ZHOU Yupeng, BAO Zhenyu. Power flow model selection method for modeling distribution system optimization problem[J]. Automation of Electric Power Systems, 2023, 47 (15): 170- 178.
DOI
|
| 14 |
LEHMANN K, GRASTIEN A, VAN HENTENRYCK P. AC-feasibility on tree networks is NP-hard[J]. IEEE Transactions on Power Systems, 2016, 31 (1): 798- 801.
DOI
|
| 15 |
YANG Z F, XIE K G, YU J, et al. A general formulation of linear power flow models: basic theory and error analysis[J]. IEEE Transactions on Power Systems, 2019, 34 (2): 1315- 1324.
DOI
|
| 16 |
向明旭, 杨知方, 余娟, 等. 配电网线性潮流模型通式及误差分析[J]. 中国电机工程学报, 2021, 41 (6): 2053- 2063, 13.
|
|
XIANG Mingxu, YANG Zhifang, YU Juan, et al. Linear power flow model in distribution network: unified expression and error analysis[J]. Proceedings of the CSEE, 2021, 41 (6): 2053- 2063, 13.
|
| 17 |
别朝红, 王锡凡. 雅可比矩阵的奇异性检测和网络孤岛的判断[J]. 电力系统自动化, 1998, 22 (11): 24- 27.
DOI
|
|
BIE Zhaohong, WANG Xifan. Detection of Jacobian singularity and network islanding[J]. Automation of Electric Power Systems, 1998, 22 (11): 24- 27.
DOI
|
| 18 |
FAN Z X, YANG Z F, YU J. Error bound restriction of linear power flow model[J]. IEEE Transactions on Power Systems, 2022, 37 (1): 808- 811.
DOI
|
| 19 |
GARCES A. A linear three-phase load flow for power distribution systems[J]. IEEE Transactions on Power Systems, 2016, 31 (1): 827- 828.
DOI
|
| 20 |
YUAN Z. Formulations and approximations of branch flow model for general power networks[J]. Journal of Modern Power Systems and Clean Energy, 2022, 10 (5): 1110- 1126.
DOI
|
| 21 |
CHEN J Q, WU W C, ROALD L A. Data-driven piecewise linearization for distribution three-phase stochastic power flow[J]. IEEE Transactions on Smart Grid, 2022, 13 (2): 1035- 1048.
DOI
|
| 22 |
LIU Y X, ZHANG N, WANG Y, et al. Data-driven power flow linearization: a regression approach[J]. IEEE Transactions on Smart Grid, 2019, 10 (3): 2569- 2580.
DOI
|
| 23 |
LI P H, WU W C, WANG X M, et al. A data-driven linear optimal power flow model for distribution networks[J]. IEEE Transactions on Power Systems, 2023, 38 (1): 956- 959.
DOI
|
| 24 |
LIU Y T, LI Z S, SUN S M. A data-driven method for online constructing linear power flow model[J]. IEEE Transactions on Industry Applications, 2023, 59 (5): 5411- 5419.
DOI
|
| 25 |
SHAO Z T, ZHAI Q Z, WU J, et al. Data based linear power flow model: investigation of a least-squares based approximation[J]. IEEE Transactions on Power Systems, 2021, 36 (5): 4246- 4258.
DOI
|
| 26 |
TAN Y, CHEN Y Y, LI Y, et al. Linearizing power flow model: a hybrid physical model-driven and data-driven approach[J]. IEEE Transactions on Power Systems, 2020, 35 (3): 2475- 2478.
DOI
|
| 27 |
巨云涛, 杨明友, 吴文传. 适用于配电网三相优化潮流的数据物理融合驱动线性化方法[J]. 电力系统自动化, 2022, 46 (13): 43- 52.
DOI
|
|
JU Yuntao, YANG Mingyou, WU Wenchuan. Hybrid data-physical-driven linearization method for three-phase optimal power flow in distribution network[J]. Automation of Electric Power Systems, 2022, 46 (13): 43- 52.
DOI
|
| 28 |
BAZRAFSHAN M, GATSIS N. Comprehensive modeling of three-phase distribution systems via the bus admittance matrix[J]. IEEE Transactions on Power Systems, 2018, 33 (2): 2015- 2029.
DOI
|
| 29 |
SHAO Z T, ZHAI Q Z, GUAN X H. Physical-model-aided data-driven linear power flow model: an approach to address missing training data[J]. IEEE Transactions on Power Systems, 2023, 38 (3): 2970- 2973.
DOI
|
| 30 |
YANG J, REN G X, LUO L, et al. CNN-LSTM combined prediction algorithm for transmission line loss rate based on improved SSA[C]//2022 5th International Conference on Electronics and Electrical Engineering Technology (EEET). Beijing, China. IEEE, 2022: 83–89.
|
| 31 |
HUANG H R. Line loss prediction of distribution network based on BP neural network[J]. Scientific Programming, 2022, 2022, 6105316.
|