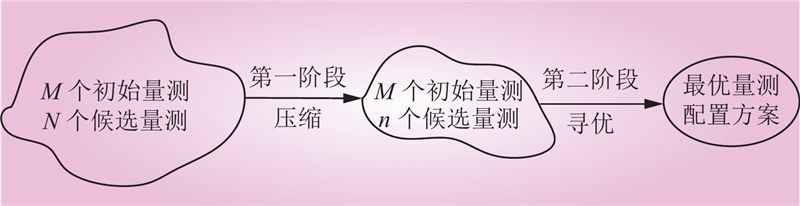
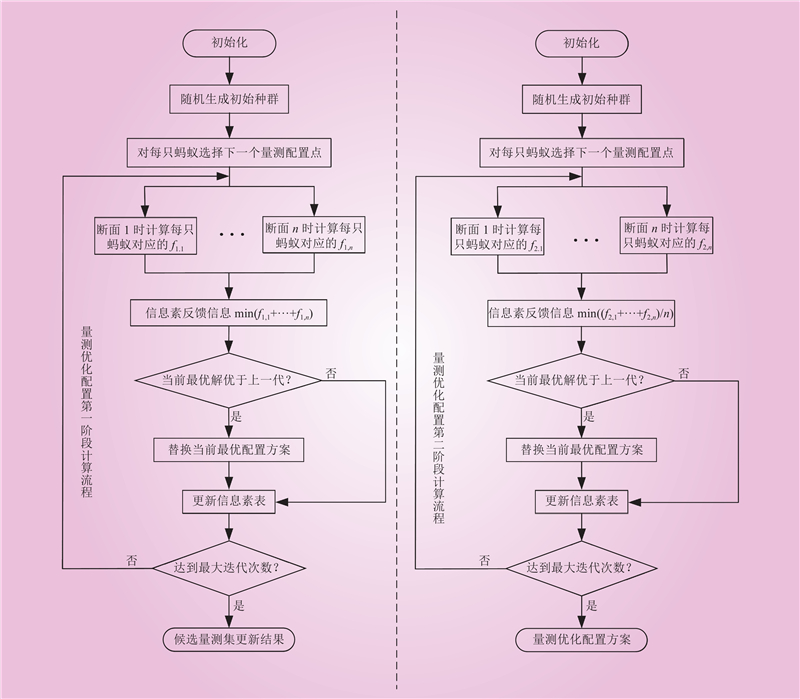
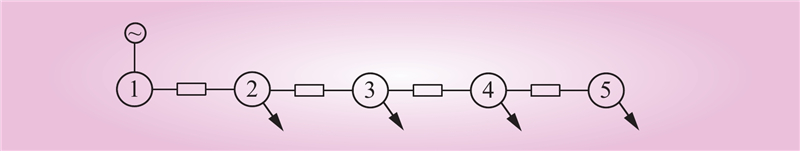
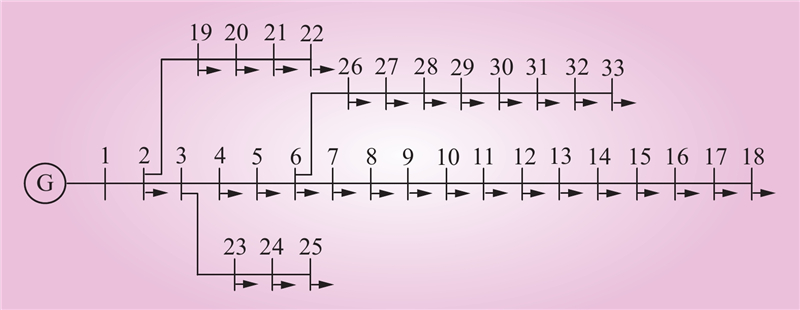
| 1 |
张子桐, 周群, 佃钰林, 等. 基于μPMU测量数据的配电网线路阻抗参数估计[J]. 中国电力, 2023, 56 (8): 157- 165.
DOI
|
|
ZHANG Zitong, ZHOU Qun, DIAN Yulin, et al. Parameter estimation for line impedance in distribution network based on μPMU data[J]. Electric Power, 2023, 56 (8): 157- 165.
DOI
|
| 2 |
程林, 万宇翔, 齐宁, 等. 含多种分布式资源的配用电系统运行可靠性研究评述及展望[J]. 电力系统自动化, 2021, 45 (22): 191- 207.
|
|
CHENG Lin, WAN Yuxiang, QI Ning, et al. Review and prospect of research on operation reliability of power distribution and consumption system considering various distributed energy resources[J]. Automation of Electric Power Systems, 2021, 45 (22): 191- 207.
|
| 3 |
MONTICELLI A, WU F F. Network observability: identification of observable islands and measurement placement[J]. IEEE Power Engineering Review, 1985, PER-5 (5): 32.
|
| 4 |
GOU B, ABUR A. An improved measurement placement algorithm for network observability[J]. IEEE Transactions on Power Systems, 2001, 16 (4): 819- 824.
DOI
|
| 5 |
GOU B, ABUR A. A direct numerical method for observability analysis[J]. IEEE Transactions on Power Systems, 2000, 15 (2): 625- 630.
DOI
|
| 6 |
尹冠雄, 王彬, 孙宏斌, 等. 多场景适配的多能流在线状态估计功能研发与应用[J]. 中国电机工程学报, 2020, 40 (21): 6794- 6804.
|
|
YIN Guanxiong, WANG Bin, SUN Hongbin, et al. Multi-scene adaptive online state estimation of multi-energy network: development and application[J]. Proceedings of the CSEE, 2020, 40 (21): 6794- 6804.
|
| 7 |
季宇, 孙彦萍, 吴鸣, 等. 基于可观测性分析的计及DG不确定性配电网表计优化配置[J]. 电力自动化设备, 2017, 37 (3): 26- 32.
|
|
JI Yu, SUN Yanping, WU Ming, et al. Optimal meter allocation based on observability analysis with consideration of DG uncertainty for distribution network[J]. Electric Power Automation Equipment, 2017, 37 (3): 26- 32.
|
| 8 |
SCHWEPPE F C, WILDES J. Power system static-state estimation, part I: exact model[J]. IEEE Transactions on Power Apparatus and Systems, 1970, PAS-89 (1): 120- 125.
DOI
|
| 9 |
徐航, 鞠力, 董树锋, 等. 提高配电网状态估计精度的智能配电单元优化布点方法[J]. 电网技术, 2018, 42 (4): 1210- 1216.
|
|
XU Hang, JU Li, DONG Shufeng, et al. Optimization of intelligent distribution unit placement to improve accuracy of distribution network state estimation[J]. Power System Technology, 2018, 42 (4): 1210- 1216.
|
| 10 |
PRASAD S, VINOD KUMAR D M. Trade-offs in PMU and IED deployment for active distribution state estimation using multi-objective evolutionary algorithm[J]. IEEE Transactions on Instrumentation and Measurement, 2018, 67 (6): 1298- 1307.
DOI
|
| 11 |
徐艳春, 刘晓明, 席磊, 等. 改进双因子抗差贝叶斯估计在区域配网状态估计中的性能分析[J]. 中国电机工程学报, 2021, 41 (14): 4879- 4890.
|
|
XU Yanchun, LIU Xiaoming, XI Lei, et al. Performance analysis of improved two-factor robust Bayes estimation in state estimation of regional distribution network[J]. Proceedings of the CSEE, 2021, 41 (14): 4879- 4890.
|
| 12 |
徐俊俊, 戴桂木, 吴在军, 等. 计及电动汽车和光伏不确定性的主动配电网量测优化配置[J]. 电力系统自动化, 2017, 41 (1): 57- 64.
DOI
|
|
XU Junjun, DAI Guimu, WU Zaijun, et al. Optimal meter placement for active distribution network considering uncertainties of plug-in electric vehicles and photovoltaic systems[J]. Automation of Electric Power Systems, 2017, 41 (1): 57- 64.
DOI
|
| 13 |
王克英, 穆钢, 陈学允. 计及PMU的状态估计精度分析及配置研究[J]. 中国电机工程学报, 2001, 21 (8): 29- 33.
DOI
|
|
WANG Keying, MU Gang, CHEN Xueyun. Precision improvement and pmu placement studies on state estimation of a hybrid measurement system with pmus[J]. Proceedings of the CSEE, 2001, 21 (8): 29- 33.
DOI
|
| 14 |
高亚静, 张占龙, 吴文传, 等. 配电网量测配置评估及优化[J]. 中国电力, 2014, 47 (7): 39- 44.
|
|
GAO Yajing, ZHANG Zhanlong, WU Wenchuan, et al. Evaluation and optimization of measurement configuration in distribution system[J]. Electric Power, 2014, 47 (7): 39- 44.
|
| 15 |
徐臣, 余贻鑫. 提高配电网状态估计精度的量测配置优化方法[J]. 电力自动化设备, 2009, 29 (7): 17- 21.
|
|
XU Chen, YU Yixin. Evaluation and optimization of meter placement to enhance distribution state estimation[J]. Electric Power Automation Equipment, 2009, 29 (7): 17- 21.
|
| 16 |
KEKATOS V, GIANNAKIS G B, WOLLENBERG B. Optimal placement of phasor measurement units via convex relaxation[J]. IEEE Transactions on Power Systems, 2012, 27 (3): 1521- 1530.
DOI
|
| 17 |
LI Q, CUI T, WENG Y, et al. An information-theoretic approach to PMU placement in electric power systems[J]. IEEE Transactions on Smart Grid, 2013, 4 (1): 446- 456.
DOI
|
| 18 |
赵媛媛, 袁澎, 艾芊, 等. 考虑拓扑约束并采用改进遗传算法的PMU优化配置[J]. 电网技术, 2014, 38 (8): 2063- 2070.
|
|
ZHAO Yuanyuan, YUAN Peng, AI Qian, et al. Improved genetic algorithm based optimal configuration of PMUs considering topological constraints[J]. Power System Technology, 2014, 38 (8): 2063- 2070.
|
| 19 |
CHEN X S, LIN J, WAN C, et al. Optimal meter placement for distribution network state estimation: a circuit representation based MILP approach[J]. IEEE Transactions on Power Systems, 2016, 31 (6): 4357- 4370.
DOI
|
| 20 |
XYGKIS T C, KORRES G N, MANOUSAKIS N M. Fisher information-based meter placement in distribution grids via the D-optimal experimental design[J]. IEEE Transactions on Smart Grid, 2018, 9 (2): 1452- 1461.
DOI
|
| 21 |
LIU J Q, PONCI F, MONTI A, et al. Optimal meter placement for robust measurement systems in active distribution grids[J]. IEEE Transactions on Instrumentation and Measurement, 2014, 63 (5): 1096- 1105.
DOI
|
| 22 |
AMINIFAR F, KHODAEI A, FOTUHI-FIRUZABAD M, et al. Contingency-constrained PMU placement in power networks[J]. IEEE Transactions on Power Systems, 2010, 25 (1): 516- 523.
DOI
|
| 23 |
张健磊, 高湛军, 王志远, 等. 基于有限μPMU的主动配电网故障定位方法[J]. 电网技术, 2020, 44 (7): 2722- 2731.
|
|
ZHANG Jianlei, GAO Zhanjun, WANG Zhiyuan, et al. Fault location method for active distribution based on finite μPMU[J]. Power System Technology, 2020, 44 (7): 2722- 2731.
|
| 24 |
COSER J, COSTA A S, ROLIM J G. Metering scheme optimization with emphasis on ensuring bad-data processing capability[J]. IEEE Transactions on Power Systems, 2006, 21 (4): 1903- 1911.
DOI
|
| 25 |
何胜, 杨斌, 俞明, 等. 一种谐波量测点优化配置的两阶段算法[J]. 电力系统及其自动化学报, 2021, 33 (6): 22- 27.
DOI
|
|
HE Sheng, YANG Bin, YU Ming, et al. Two-stage algorithm for optimal configuration of harmonic measurement points[J]. Proceedings of the CSU-EPSA, 2021, 33 (6): 22- 27.
DOI
|
| 26 |
孙国城, 赵祖康. 地区电网状态估计研究[J]. 电力系统自动化, 1993, 17 (12): 41- 45.
DOI
|
|
SUN Guocheng, ZHAO Zukang. State estimation research on electrical distribution system[J]. Automation of Electric Power Systems, 1993, 17 (12): 41- 45.
DOI
|
| 27 |
SEBERRY J , YAMADA M . More on maximal determinant matrices[M]. Hadamard Matrices: Constructions using Number Theory and Linear Algebra. Wiley: 245–269.
|
| 28 |
BU F K, YUAN Y X, WANG Z Y, et al. A time-series distribution test system based on real utility data[C]//2019 North American Power Symposium (NAPS). Wichita, KS, USA. IEEE, 2020: 1–6.
|