电热综合能源系统稳态与区间潮流计算快速解耦新方法

doi:10.11930/j.issn.1004-9649.202309019

中国电力 ›› 2024, Vol. 57 ›› Issue (7): 203-213.DOI: 10.11930/j.issn.1004-9649.202309019

电热综合能源系统稳态与区间潮流计算快速解耦新方法

景巍巍¹(), 王强², 程好³, 王博¹(), 岳付昌¹(), 王沉⁴(), 王文学¹()

1. 国网江苏省电力有限公司连云港供电分公司，江苏连云港　222000
2. 江苏齐天电力建设集团有限公司，江苏连云港　222000
3. 连云港智源电力设计有限公司，江苏连云港　222000
4. 河海大学电气与动力工程学院，江苏南京　211100

收稿日期:2023-09-05 出版日期:2024-07-28 发布日期:2024-07-23
作者简介:景巍巍（1981—），男，高级工程师，从事综合能源系统运行与优化、电力系统调度与运行控制研究，E-mail：jony_jing@163.com
王博（1988—），男，高级工程师，从事电力系统调度与运行控制研究，E-mail：feiwu20060603@126.com
岳付昌（1983—），男，高级工程师，从事电力系统运行与控制研究，E-mail：345591160@qq.com
王沉（1999—），男，硕士研究生，从事综合能源系统运行与优化研究，E-mail：2794764948@qq.com
王文学（1993—），男，通信作者，中级工程师，从事综合能源系统运行与优化研究，E-mail：767886650@qq.com
基金资助:
国家自然科学基金资助项目（51877071）；中央高校基本科研业务费项目（B220202006）；江苏省沿海电力基础设施智能化工程研究中心科技项目。

New Rapid Decoupling Methods for Calculating Steady-State and Interval Power Flow of Integrated Electricity-Heat Energy Systems

Weiwei JING¹(), Qiang WANG², Hao CHENG³, Bo WANG¹(), Fuchang YUE¹(), Chen WANG⁴(), Wenxue WANG¹()

1. Lianyungang Power Supply Company of State Grid Jiangsu Electric Power Co., Ltd., Lianyungang 222000, China
2. Jiangsu Qitian Electric Power Construction Group Co., Ltd., Lianyungang 222000, China
3. Lianyungang Zhiyuan Electric Power Design Co., Ltd., Lianyungang 222000, China
4. School of Electrical and Power Engineering, Hohai University, Nanjing 211100, China

Received:2023-09-05 Online:2024-07-28 Published:2024-07-23
Supported by:
This work is supported by National Natural Science Foundation of China (No.51877071), Fundamental Research Funds for the Central Universities (No.B220202006) and Jiangsu Coastal Power Infrastructure Intelligent Engineering Research Center Science and Technology Project.

摘要/Abstract

摘要：

综合能源系统稳态潮流计算是后续规划、运行等研究的基础。目前常采用的牛顿-拉夫逊法存在数值稳定性问题，且随着综合能源系统不确定因素增加，研究系统的区间潮流以实现综合能源系统的安全分析和评估变得越来越重要。首先，基于泰勒函数一阶展开和二阶展开，推导出热负荷流量和节点温度的解析表达式，该表达式实现了流量与温度的独立求解，无须解方程组即可得到系统稳态潮流。然后，分析流量和温度表达式的函数单调性，结合各热负荷值，确定了热负荷流量和热负荷节点温度的区间潮流解，所提方法在保证计算精度的同时，计算速度快且不存在数值稳定性问题。最后，通过算例分析验证了所提方法的有效性。

关键词: 电-热互联综合能源系统, 稳态潮流, 区间潮流, 解耦计算, 解析式方法

Abstract:

Steady-state power flow calculation of integrated energy systems is fundamental to subsequent planning and operational studies. The commonly used Newton-Raphson method poses challenges in numerical stability. Moreover, with the increasing uncertainty in integrated energy systems, it becomes increasingly vital to study the interval power flows for the safety analysis and evaluation of the integrated energy systems. Initially, based on the first and second order expansions of the Taylor function, the analytical expressions for heat load flow and nodal temperature are derived, which allow for the independent solution of flow rate and temperature, enabling the determination of system steady-state flow without solving equation set. And then, the monotonicity of the flow rate and temperature expressions is analyzed in conjunction with heat load values to determine the interval flow solutions for heat load flow and nodal temperature. The proposed method is fast in calculation speed and free from numerical stability problems while ensuring the computational accuracy. Finally, the effectiveness of the proposed method is validated through case study analysis.

Key words: electric-thermal interconnected integrated energy system, steady-state power flow, interval power flow, decoupling calculation, analytical method

景巍巍, 王强, 程好, 王博, 岳付昌, 王沉, 王文学. 电热综合能源系统稳态与区间潮流计算快速解耦新方法[J]. 中国电力, 2024, 57(7): 203-213.

Weiwei JING, Qiang WANG, Hao CHENG, Bo WANG, Fuchang YUE, Chen WANG, Wenxue WANG. New Rapid Decoupling Methods for Calculating Steady-State and Interval Power Flow of Integrated Electricity-Heat Energy Systems[J]. Electric Power, 2024, 57(7): 203-213.

导出引用管理器 EndNote|Ris|BibTeX

链接本文: https://www.electricpower.com.cn/CN/10.11930/j.issn.1004-9649.202309019

https://www.electricpower.com.cn/CN/Y2024/V57/I7/203

图/表 18

参考文献 25

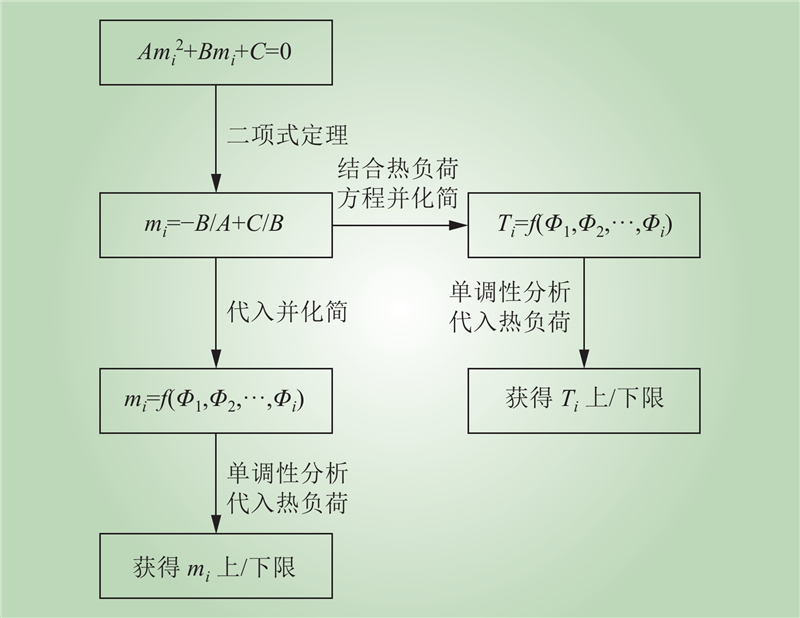
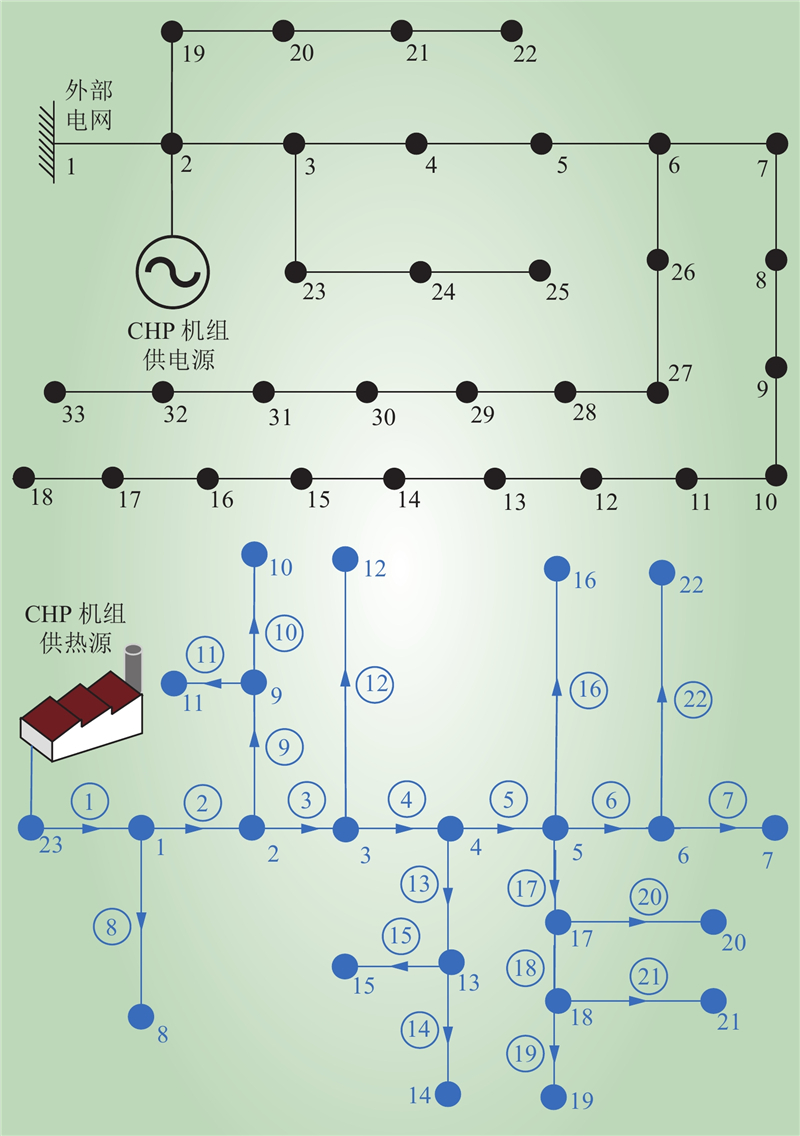
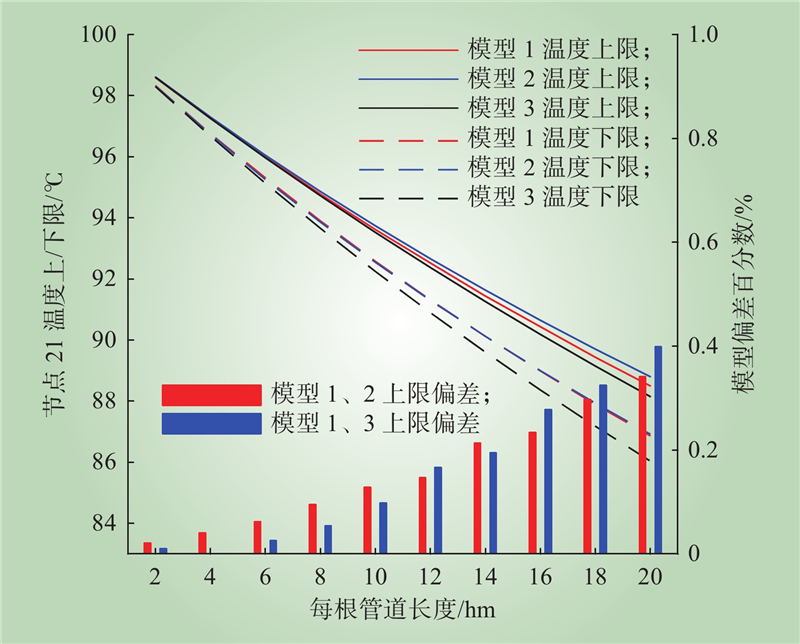
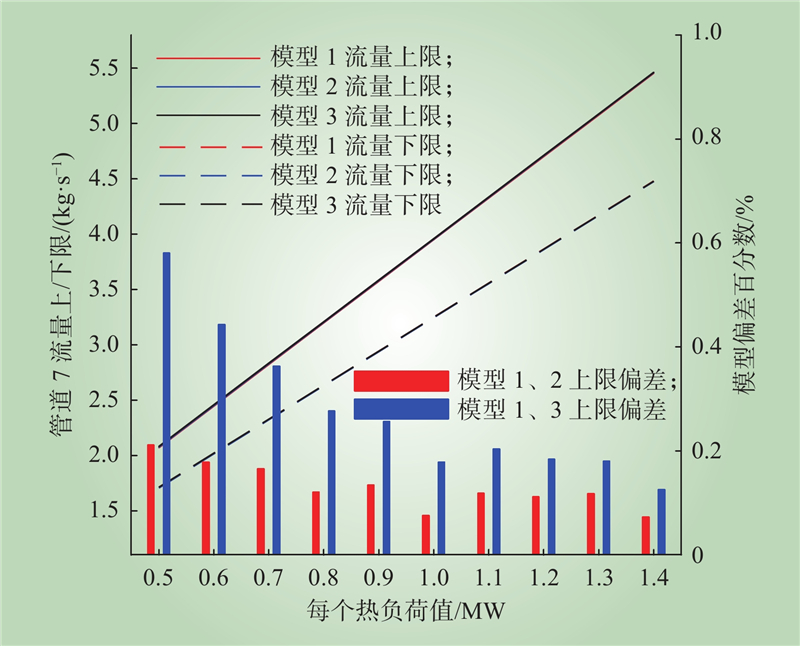
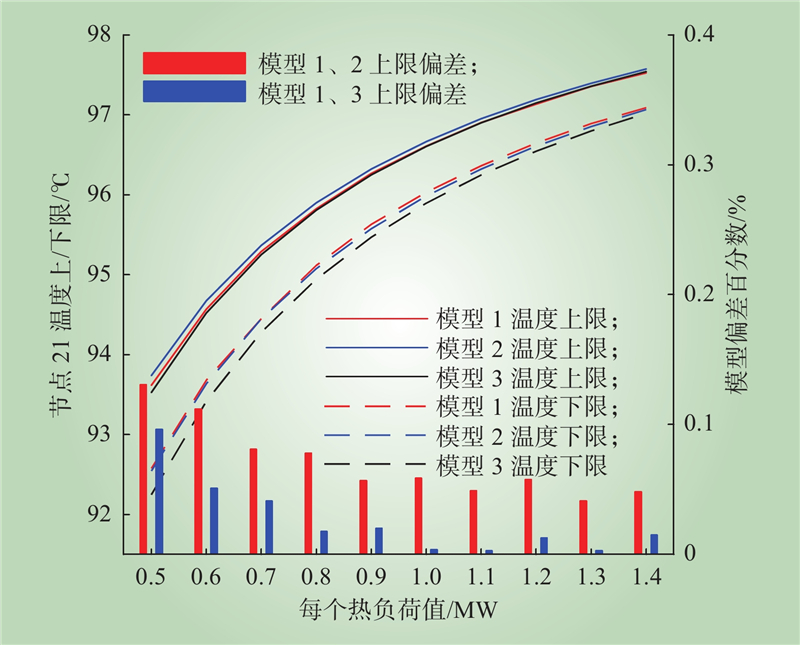
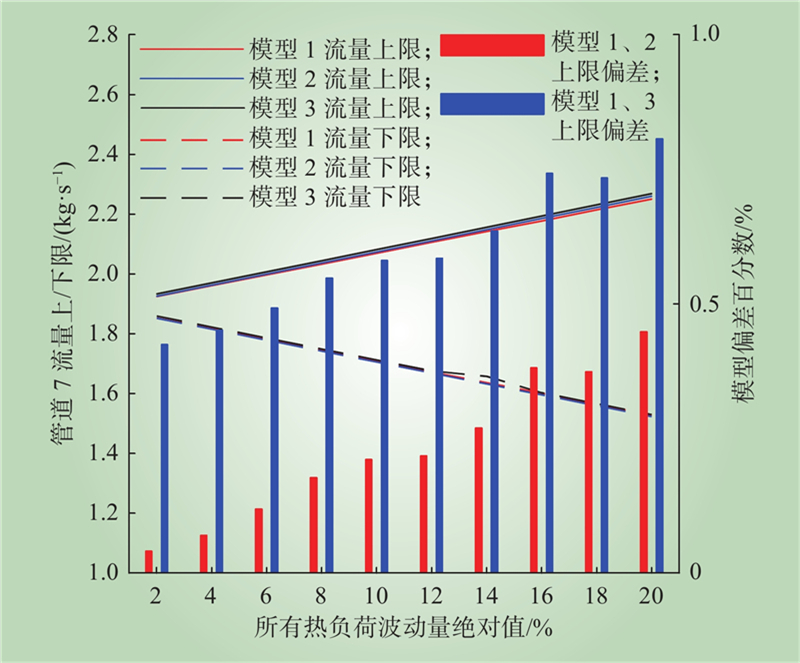
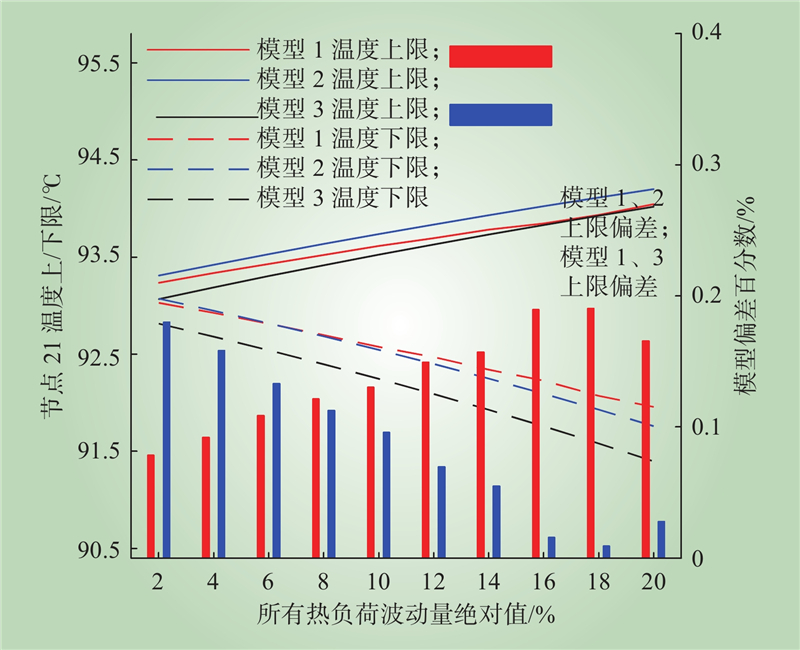
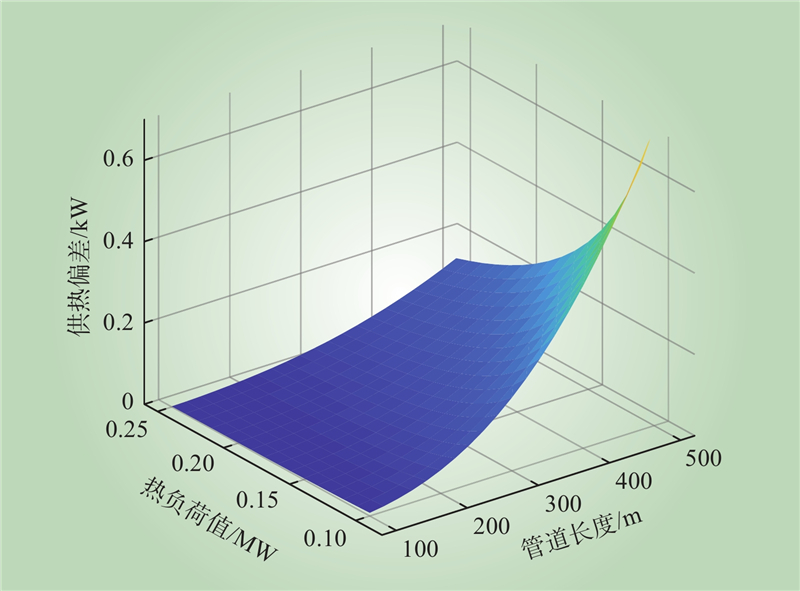
1	SHABANPOUR-HAGHIGHI A, SEIFI A R. An integrated steady-state operation assessment of electrical, natural gas, and district heating networks[J]. IEEE Transactions on Power Systems, 2016, 31 (5): 3636- 3647. DOI
2	MASSRUR H R, NIKNAM T, AGHAEI J, et al. Fast decomposed energy flow in large-scale integrated electricity-gas-heat energy systems[J]. IEEE Transactions on Sustainable Energy, 2018, 9 (4): 1565- 1577. DOI
3	MAGDY G, MOHAMED E A, SHABIB G, et al. Microgrid dynamic security considering high penetration of renewable energy[J]. Protection and Control of Modern Power Systems, 2018, 3 (3): 1- 11.
4	王伟亮, 王丹, 贾宏杰, 等. 能源互联网背景下的典型区域综合能源系统稳态分析研究综述[J]. 中国电机工程学报, 2016, 36 (12): 3292- 3305.
	WANG Weiliang, WANG Dan, JIA Hongjie, et al. Review of steady-state analysis of typical regional integrated energy system under the background of energy Internet[J]. Proceedings of the CSEE, 2016, 36 (12): 3292- 3305.
5	LIU X Z. Combined analysis of electricity and heat networks[D]. Cardiff, Wales, UK: Cardiff University, 2013.
6	LIU X Z, WU J Z, JENKINS N, et al. Combined analysis of electricity and heat networks[J]. Applied Energy, 2016, 162, 1238- 1250. DOI
7	刘述欣, 戴赛, 胡林献, 等. 计及回水管网热损失的电热联合系统潮流模型及算法[J]. 电力系统自动化, 2018, 42 (4): 77- 81. DOI
	LIU Shuxin, DAI Sai, HU Linxian, et al. Power flow model and algorithm of combined power and heat system considering heat loss in return pipe network[J]. Automation of Electric Power Systems, 2018, 42 (4): 77- 81. DOI
8	顾伟, 陆帅, 王珺, 等. 多区域综合能源系统热网建模及系统运行优化[J]. 中国电机工程学报, 2017, 37 (5): 1305- 1316.
	GU Wei, LU Shuai, WANG Jun, et al. Modeling of the heating network for multi-district integrated energy system and its operation optimization[J]. Proceedings of the CSEE, 2017, 37 (5): 1305- 1316.
9	王英瑞, 曾博, 郭经, 等. 电–热–气综合能源系统多能流计算方法[J]. 电网技术, 2016, 40 (10): 2942- 2951.
	WANG Yingrui, ZENG Bo, GUO Jing, et al. Multi-energy flow calculation method for integrated energy system containing electricity, heat and gas[J]. Power System Technology, 2016, 40 (10): 2942- 2951.
10	徐宪东, 贾宏杰, 靳小龙, 等. 区域综合能源系统电/气/热混合潮流算法研究[J]. 中国电机工程学报, 2015, 35 (14): 3634- 3642.
	XU Xiandong, JIA Hongjie, JIN Xiaolong, et al. Study on hybrid heat-gas-power flow algorithm for integrated community energy system[J]. Proceedings of the CSEE, 2015, 35 (14): 3634- 3642.
11	CHEN Y B, ZHAO J Y, MA J. Fast decoupled multi-energy flow calculation for integrated energy system[J]. Journal of Modern Power Systems and Clean Energy, 2020, 8 (5): 951- 960. DOI
12	陈彬彬, 孙宏斌, 尹冠雄, 等. 综合能源系统分析的统一能路理论(二): 水路与热路[J]. 中国电机工程学报, 2020, 40 (7): 2133- 2142, 2393.
	CHEN Binbin, SUN Hongbin, YIN Guanxiong, et al. Energy circuit theory of integrated energy system analysis(II): hydraulic circuit and thermal circuit[J]. Proceedings of the CSEE, 2020, 40 (7): 2133- 2142, 2393.
13	陈彬彬, 孙宏斌, 吴文传, 等. 综合能源系统分析的统一能路理论(三): 稳态与动态潮流计算[J]. 中国电机工程学报, 2020, 40 (15): 4820- 4831.
	CHEN Binbin, SUN Hongbin, WU Wenchuan, et al. Energy circuit theory of integrated energy system analysis(Ⅲ): steady and dynamic energy flow calculation[J]. Proceedings of the CSEE, 2020, 40 (15): 4820- 4831.
14	胡枭, 尚策, 程浩忠, 等. 综合能源系统能流计算方法述评与展望[J]. 电力系统自动化, 2020, 44 (18): 179- 191.
	HU Xiao, SHANG Ce, CHENG Haozhong, et al. Review and prospect of calculation method for energy flow in integrated energy system[J]. Automation of Electric Power Systems, 2020, 44 (18): 179- 191.
15	卢炳文, 魏震波, 魏平桉, 等. 考虑多重区间不确定性的用户侧综合能源系统双层优化配置[J]. 中国电力, 2022, 55 (3): 193- 202.
	LU Bingwen, WEI Zhenbo, WEI Pingan, et al. Two-level optimal configuration of user-side integrated energy system considering interval uncertainties[J]. Electric Power, 2022, 55 (3): 193- 202.
16	郭祚刚, 徐敏, 于浩, 等. 考虑多重不确定性的园区综合能源系统区间优化调度[J]. 中国电力, 2022, 55 (11): 121- 128, 141.
	GUO Zuogang, XU Min, YU Hao, et al. Interval optimal dispatching of community integrated energy system considering multiple uncertainties[J]. Electric Power, 2022, 55 (11): 121- 128, 141.
17	伍惠铖, 王淳, 刘宽, 等. 电-热综合能源系统能流的区间计算算法[J]. 电网技术, 2019, 43 (1): 91- 99.
	WU Huicheng, WANG Chun, LIU Kuan, et al. An interval energy flow calculation method for integrated electro-thermal energy system[J]. Power System Technology, 2019, 43 (1): 91- 99.
18	王文学, 胡伟, 孙国强, 等. 电-热互联综合能源系统区间潮流计算方法[J]. 电网技术, 2019, 43 (1): 83- 95.
	WANG Wenxue, HU Wei, SUN Guoqiang, et al. Interval energy flow calculation method of integrated electro-thermal system[J]. Power System Technology, 2019, 43 (1): 83- 95.
19	张涛, 刘伉, 陶然, 等. 计及热媒流率和热损耗不确定性的综合能源系统优化调度[J]. 中国电力, 2023, 56 (4): 146- 155.
	ZHANG Tao, LIU Kang, TAO Ran, et al. Optimal scheduling of integrated energy system considering uncertainty of heat medium flow rate and heating network loss[J]. Electric Power, 2023, 56 (4): 146- 155.
20	陈胜, 卫志农, 孙国强, 等. 电-气混联综合能源系统概率能量流分析[J]. 中国电机工程学报, 2015, 35 (24): 6331- 6340.
	CHEN Sheng, WEI Zhinong, SUN Guoqiang, et al. Probabilistic energy flow analysis in integrated electricity and natural-gas energy systems[J]. Proceedings of the CSEE, 2015, 35 (24): 6331- 6340.
21	韩佶, 苗世洪, 李超, 等. 计及相关性的电-气-热综合能源系统概率最优能量流[J]. 电工技术学报, 2019, 34 (5): 1055- 1067.
	HAN Ji, MIAO Shihong, LI Chao, et al. Probabilistic optimal energy flow of electricity-gas-heat integrated energy system considering correlation[J]. Transactions of China Electrotechnical Society, 2019, 34 (5): 1055- 1067.
22	李红, 王文学, 伏祥运, 等. 基于解析法的电-热互联综合能源系统概率潮流计算[J]. 电力工程技术, 2021, 40 (5): 151- 157.
	LI Hong, WANG Wenxue, FU Xiangyun, et al. Probability power flow calculation for electric-thermal interconnected integrated energy system based on analytical method[J]. Electric Power Engineering Technology, 2021, 40 (5): 151- 157.
23	孙国强, 王文学, 吴奕, 等. 辐射型电–热互联综合能源系统快速潮流计算方法[J]. 中国电机工程学报, 2020, 40 (13): 4131- 4142.
	SUN Guoqiang, WANG Wenxue, WU Yi, et al. Fast power flow calculation method for radiant electric-thermal interconnected integrated energy system[J]. Proceedings of the CSEE, 2020, 40 (13): 4131- 4142.
24	张敏, 王金浩, 常潇, 等. 考虑可再生能源不确定性的热-电耦合微能源系统多目标鲁棒规划方法[J]. 中国电力, 2021, 54 (4): 119- 129, 140.
	ZHANG Min, WANG Jinhao, CHANG Xiao, et al. A multi-objective robust planning method for thermal-electrical coupling micro-energy system considering the uncertainty of renewable energy[J]. Electric Power, 2021, 54 (4): 119- 129, 140.
25	罗金满, 赵善龙, 封祐钧, 等. 考虑综合需求响应不确定性的电-气综合能源系统优化运行[J]. 中国电力, 2020, 53 (12): 119- 126.
	LUO Jinman, ZHAO Shanlong, FENG Youjun, et al. Optimal operation of integrated electricity-gas system considering uncertainty of integrated demand response[J]. Electric Power, 2020, 53 (12): 119- 126.

管道编号	管道流量/(kg·s^–1)			偏差/%		流量均方根误差/ (kg·s^–1)		流量平均绝对误差/ (kg·s^–1)
管道编号	模型1	模型2	模型3	模型 1、2	模型 1、3	模型 1、2	模型 1、3	模型 1、2	模型 1、3
7	1.8007	1.8003	1.8022	0.0222	0.0833	2.06× 10^–4	39.3× 10^–4	1.58× 10^–4	10.8× 10^–4
8	1.7440	1.7441	1.7444	0.0057	0.0229
10	1.7668	1.7669	1.7676	0.0057	0.0453
11	1.7642	1.7643	1.7650	0.0057	0.0453
12	1.7561	1.7563	1.7568	0.0114	0.0399
14	1.7820	1.7820	1.7832	0.0000	0.0673
15	1.7775	1.7775	1.7786	0.0000	0.0619
16	1.7645	1.7647	1.7655	0.0113	0.0567
19	1.7950	1.7948	1.7965	0.0111	0.0836
20	1.7739	1.7741	1.7751	0.0113	0.0676
21	1.8022	1.8018	1.8037	0.0222	0.0832
22	1.7823	1.7823	1.7836	0.0000	0.0729

管道编号	管道流量/(kg·s^–1)			偏差/%		流量均方根误差/ (kg·s^–1)		流量平均绝对误差/ (kg·s^–1)
管道编号	模型1	模型2	模型3	模型 1、2	模型 1、3	模型 1、2	模型 1、3	模型 1、2	模型 1、3
7	1.8007	1.8003	1.8022	0.0222	0.0833	2.06× 10^–4	39.3× 10^–4	1.58× 10^–4	10.8× 10^–4
8	1.7440	1.7441	1.7444	0.0057	0.0229
10	1.7668	1.7669	1.7676	0.0057	0.0453
11	1.7642	1.7643	1.7650	0.0057	0.0453
12	1.7561	1.7563	1.7568	0.0114	0.0399
14	1.7820	1.7820	1.7832	0.0000	0.0673
15	1.7775	1.7775	1.7786	0.0000	0.0619
16	1.7645	1.7647	1.7655	0.0113	0.0567
19	1.7950	1.7948	1.7965	0.0111	0.0836
20	1.7739	1.7741	1.7751	0.0113	0.0676
21	1.8022	1.8018	1.8037	0.0222	0.0832
22	1.7823	1.7823	1.7836	0.0000	0.0729

节点编号	供水温度/℃			偏差/%
节点编号	模型1	模型2	模型3	模型1、2	模型1、3
7	96.3975	96.4120	96.3414	0.0150	0.0582
8	98.5540	98.5512	98.5396	0.0028	0.0146
10	97.6696	97.6684	97.6385	0.0012	0.0318
11	97.7687	97.7661	97.7386	0.0027	0.0308
12	98.0831	98.0765	98.0561	0.0067	0.0275
14	97.0921	97.0941	97.0477	0.0021	0.0457
15	97.2647	97.2628	97.2216	0.0020	0.0443
16	97.7604	97.7499	97.7220	0.0107	0.0393
19	96.6070	96.6143	96.5515	0.0076	0.0574
20	97.3979	97.3900	97.3525	0.0081	0.0466
21	96.3417	96.3576	96.2849	0.0165	0.0590
22	97.0831	97.0801	97.0333	0.0031	0.0513

节点编号	供水温度/℃			偏差/%
节点编号	模型1	模型2	模型3	模型1、2	模型1、3
7	96.3975	96.4120	96.3414	0.0150	0.0582
8	98.5540	98.5512	98.5396	0.0028	0.0146
10	97.6696	97.6684	97.6385	0.0012	0.0318
11	97.7687	97.7661	97.7386	0.0027	0.0308
12	98.0831	98.0765	98.0561	0.0067	0.0275
14	97.0921	97.0941	97.0477	0.0021	0.0457
15	97.2647	97.2628	97.2216	0.0020	0.0443
16	97.7604	97.7499	97.7220	0.0107	0.0393
19	96.6070	96.6143	96.5515	0.0076	0.0574
20	97.3979	97.3900	97.3525	0.0081	0.0466
21	96.3417	96.3576	96.2849	0.0165	0.0590
22	97.0831	97.0801	97.0333	0.0031	0.0513

管道编号	流量上限/(kg·s^–1)			偏差/%
管道编号	模型1	模型2	模型3	模型1、2	模型1、3
7	1.9765	1.9782	1.9802	0.0860	0.1872
8	1.9154	1.9157	1.9160	0.0157	0.0313
10	1.9403	1.9410	1.9418	0.0361	0.0773
11	1.9377	1.9384	1.9391	0.0361	0.0723
12	1.9288	1.9298	1.9303	0.0518	0.0778
14	1.9568	1.9588	1.9601	0.1022	0.1686
15	1.9525	1.9543	1.9555	0.0922	0.1536
16	1.9393	1.9409	1.9416	0.0825	0.1186
19	1.9719	1.9743	1.9762	0.1217	0.2181
20	1.9496	1.9516	1.9527	0.1026	0.1590
21	1.9793	1.9812	1.9834	0.0960	0.2071
22	1.9579	1.9602	1.9616	0.1175	0.1890

电热综合能源系统稳态与区间潮流计算快速解耦新方法

New Rapid Decoupling Methods for Calculating Steady-State and Interval Power Flow of Integrated Electricity-Heat Energy Systems

RichHTML

PDF (PC)

可视化

摘要/Abstract

引用本文

使用本文

图/表 18

参考文献 25

相关文章 0

编辑推荐

Metrics

管道编号	流量下限/(kg·s^–1)			偏差/%
管道编号	模型1	模型2	模型3	模型1、2	模型1、3
7	1.6247	1.6231	1.6249	0.0985	0.0123
8	1.5728	1.5726	1.5729	0.0127	0.0064
10	1.5936	1.5930	1.5938	0.0377	0.0126
11	1.5911	1.5904	1.5912	0.0440	0.0063
12	1.5839	1.5831	1.5837	0.0505	0.0126
14	1.6070	1.6057	1.6069	0.0809	0.0062
15	1.6022	1.6013	1.6023	0.0562	0.0062
16	1.5904	1.5893	1.5900	0.0692	0.0252
19	1.6174	1.6161	1.6177	0.0804	0.0185
20	1.5988	1.5975	1.5985	0.0813	0.0188
21	1.6247	1.6231	1.6249	0.0985	0.0123
22	1.6067	1.6052	1.6063	0.0934	0.0249

管道编号	温度上限/℃			偏差/%
管道编号	模型1	模型2	模型3	模型1、2	模型1、3
7	96.6707	96.7173	96.6581	0.0482	0.0130
8	98.6761	98.6795	98.6698	0.0034	0.0064
10	97.8573	97.8715	97.8466	0.0145	0.0109
11	97.9465	97.9611	97.9381	0.0149	0.0086
12	98.2316	98.2453	98.2283	0.0139	0.0034
14	97.3169	97.3446	97.3058	0.0285	0.0114
15	97.4713	97.4994	97.4650	0.0288	0.0065
16	97.9233	97.9462	97.9229	0.0234	0.0004
19	96.8598	96.9035	96.8509	0.0451	0.0092
20	97.5855	97.6162	97.5849	0.0315	0.0006
21	96.6174	96.6672	96.6063	0.0515	0.0115
22	97.3014	97.3317	97.2925	0.0311	0.0091

节点编号	温度下限/℃			偏差/%
节点编号	模型1	模型2	模型3	模型1、2	模型1、3
7	96.0680	96.0440	95.9584	0.0250	0.1141
8	98.4075	98.3953	98.3811	0.0124	0.0268
10	97.4450	97.4225	97.3859	0.0231	0.0606
11	97.5541	97.5299	97.4963	0.0248	0.0592
12	97.9060	97.8717	97.8467	0.0350	0.0606
14	96.8342	96.7915	96.7350	0.0441	0.1024
15	97.0186	96.9766	96.9264	0.0433	0.0950
16	97.5730	97.5121	97.4780	0.0624	0.0974
19	96.3094	96.2655	96.1892	0.0456	0.1248
20	97.1731	97.1164	97.0707	0.0583	0.1054
21	96.0145	95.9845	95.8963	0.0312	0.1231
22	96.8205	96.7761	96.7191	0.0459	0.1047

模态框（Modal）标题

电热综合能源系统稳态与区间潮流计算快速解耦新方法

New Rapid Decoupling Methods for Calculating Steady-State and Interval Power Flow of Integrated Electricity-Heat Energy Systems

RichHTML

PDF (PC)

可视化

摘要/Abstract

引用本文

使用本文

图/表 18

参考文献 25

相关文章 0

编辑推荐

Metrics